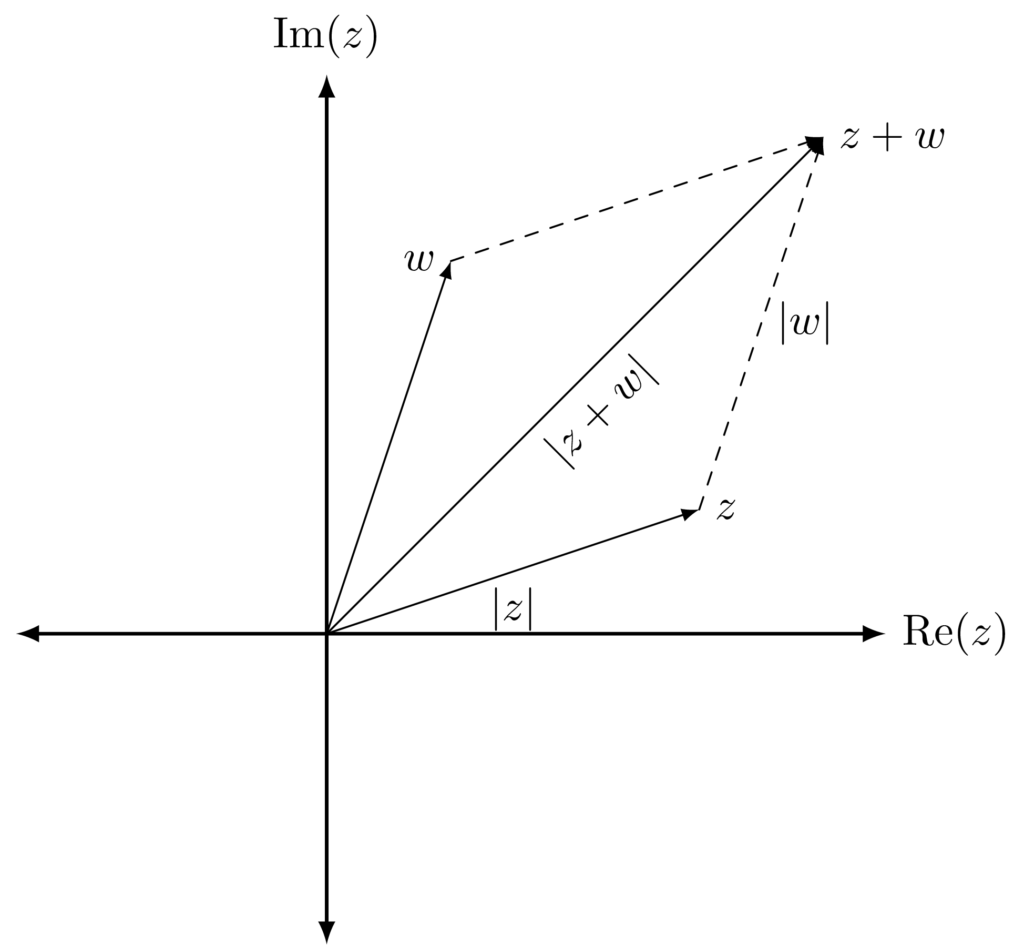
Triangle inequality. For complex numbers \(z\) and \(w\), we have $$|z|+|w| \ge |z+w|.$$
“Proof”.1 We use the fact that the sum of two sides of a triangle is longer than the third side in the below diagram.
Problems
- Show that \(|z|-|w|\ge |z-w|\) and \(|w|-|z|\ge |z-w|\), and conclude that $$\left||z|-|w|\right|\ge |z-w|.$$ This is known as the reverse triangle inequality.
- Hard. Prove the triangle inequality algebraically.